Projectile Motion

Pronunciation: /prəˈdʒɛk.taɪl ˈmoʊ.ʃən/ Explain

Projectile motion is the vertical motion of an object in a gravitational field given an initial velocity and height. The quadratic equation for projectile motion is: where t represents time, a represents acceleration due to gravity, v₀ represents the initial velocity, and h₀ represents initial height. Since down is taken to be negative, acceleration due to gravity is a negative number.

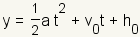
Manipulative

Click on the blue points on the sliders and drag to change the figure. What initial angle would make the projectile go only up or down?

Manipulative 1 - Projectile Motion Created with GeoGebra.

Example

1. Jeff is standing on top of 20 foot tower. He throws a stick down at an initial velocity of -10 ft/s. Use -32 ft/s² for the acceleration due to gravity. What is the equation for the vertical height of the stick? At what time does the stick reach the ground?

   Step	Equation(s)	Description
   1	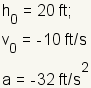	Identify the values of the constants.
   2	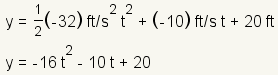	Plug the constants into the equation. Simplify the equation.
   3		Set the height to zero.
   4	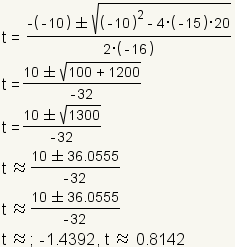	Apply the quadratic formula.
   5	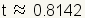	Since a negative solution does not make sense in this problem, the negative solution is extraneous solution. Discard the extraneous solution.
   Example 1

References

1. McAdams, David E.. All Math Words Dictionary, projectile motion. 2nd Classroom edition 20150108-4799968. pg 145. Life is a Story Problem LLC. January 8, 2015. Buy the book

Cite this article as:

McAdams, David E. Projectile Motion. 4/28/2019. All Math Words Encyclopedia. Life is a Story Problem LLC. http://www.allmathwords.org/en/p/projectilemotion.html.

Image Credits

• All images and manipulatives are by David McAdams unless otherwise stated. All images by David McAdams are Copyright © Life is a Story Problem LLC and are licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.

Revision History

4/28/2019: Changed equations and expressions to new format. (McAdams, David E.)
12/21/2018: Reviewed and corrected IPA pronunication. (McAdams, David E.)
12/1/2018: Removed broken links, updated license, implemented new markup, updated geogebra app. (McAdams, David E.)
8/7/2018: Changed vocabulary links to WORDLINK format. (McAdams, David E.)
1/10/2009: Initial version. (McAdams, David E.)