Rational Expression

Pronunciation: /ˈræʃ.nl ɪkˈsprɛ.ʃən/ Explain
Three rational expressions: 2/(x-1), 3+(x+2)/(x-1), (x^2-4x+2)/(x^3-5)
Figure 1: Examples of rational expressions.

A rational expression is an expression that contains one or more ratios of polynomials.

Simplifying Rational Expressions

Simplifying a rational expression is similar to reducing a fraction. Both simplifying a rational expression and reducing fractions involve finding common factors and canceling those factors.

Example 1

StepEquationDescription
1(x^2+5x+6)/(x^2+3x-3) This is the rational expression to simplify.
2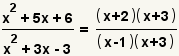(x^2+5x+6)/(x^2+3x-3)=((x+2)(x+3))/((x-1)(x+3)) Factor both of the polynomials.
3((x+2)(x+3))/((x-1)(x+3))=(x+2)/(x-1), x!=-3 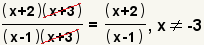Cancel common factors and place any restrictions on the resulting expression.
Example 1

Example 2

StepEquationDescription
12+(3x^2-2x-8)/(x-2) This is the rational expression to simplify.
22/1+(3x^2-2x-8)/(x-2) Transform the 2 to a fraction in order to find the common denominator.
3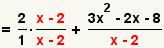(2/1)*((x-2)/(x-2))+(3x^2-2x-8)/(x-2) Multiply the 2 by ( x - 2 ) / ( x - 2 ) so the fractions can be combined.
4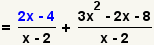(2x-4)/(x-2)+(3x^2-2x-8)/(x-2) Multiply the first two fractions.
5(2x-4+3x^2-2x-8)/(x-2) As the fractions now have a common denominator, combine the fractions.
6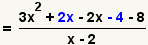(3x^2+2x-2x-4-8)/(x-2) Use the commutative law of addition to put like terms next to each other.
7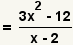(3x^2-12)/(x-2) Combine like terms.
8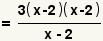(3(x-2)(x-2))/(x-2) Factor the numerator and the denominator.
9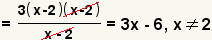(3(x-2)(x-2))/(x-2)=3(x-2),x!=2 Cancel common factors and place any restrictions on the resulting expression.
Example 2

Addition and Subtraction of Rational Expressions

Two or more rational expressions can added or subtracted. Addition and subtraction of rational expressions is similar to addition and subtraction of fractions: The steps are:

  • Find the least common denominator.
  • Transform both rational expressions to the same common denominator.
  • Add or subtract the rational expressions by adding or subtracting the numerators and using the least common denominator.

Example 3

StepEquationDescription
1x/(x-1) + x/(x+1)This is the original expression.
2The prime factorization of x - 1 is x - 1. To find the common denominator, find the prime factorization of the denominator of the first expression.
3 The prime factorization of x + 1 is x + 1. Find the prime factorization of the denominator of the second expression.
4 The factor x + 1 is not found in the second denominator. The second rational expression will be multiplied by 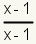(x+1)/(x+1). Identify the factors of the second denominator not in the first denominator. The first rational expression will be multiplied by a ratio of these factors.
5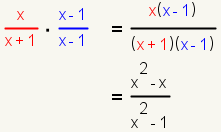(x/(x+1))*((x-1)/(x-1))=(x(x-1))/((x+1)(x-1))=(x^2-x)/(x^2-1) Multiply the first rational expression by the factors identified in step 4.
6 The factor x - 1 is not found in the first denominator. The first rational expression will be multiplied by 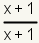(x+1)/(x+1). Identify the factors of the first denominator not in the second denominator. The second rational expression will be multiplied by a ratio of these factors.
7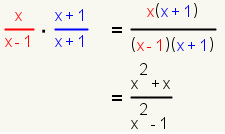(x/(x-1))*((x+1)/(x+1))=(x(x+1))/((x-1)(x+1))=(x^2+x)/(x^2-1) Multiply the second rational expression by the factors identified in step 6.
8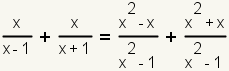x/(x-1) + x/(x+1) = (x^2-x)/(x^2-1) + (x^2+x)/(x^2+1) Transform the expressions using the multiplied rational expressions.
9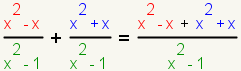((x^2-x)/(x^2-1)) + ((x^2+x)/(x^2-1)) = (x^2 - x + x^2 + x)/(x^2 - 1) Add the numerators, and copy the denominator.
10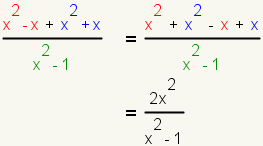(x^2 - x + x^2 + x)/(x^2 - 1) = (x^2 + x^2 - x + x)/(x^2 - 1) = (2x^2)/(x^2-1) Simplify the numerator by combining like terms.
11x/(x-1) + x/(x+1) = (2x^2)/(x^2-1) This is the answer.
Example 3 - Addition of rational expressions

Example 4

StepEquationDescription
11/x - (x-2)/(x^2 + x) This is the original expression.
2 The prime factorization of x is x. To find the common denominator, find the prime factorization of the denominator of the first expression.
3 The prime factorization of x2 + x is x( x + 1 ). Find the prime factorization of the denominator of the second expression.
4The factor x is found in the second denominator. The second rational expression is already common. Identify the factors of the second denominator not in the first denominator. The first rational expression will be multiplied by a ratio of these factors.
5The factor x is found in the first denominator. The factor x + 1 is not found in the first denominator. The first rational expression will be multiplied by 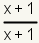(x+1)/(x+1). Identify the factors of the first denominator not in the second denominator. The second rational expression will be multiplied by a ratio of these factors.
6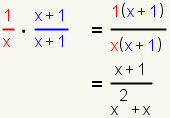(1/x)*((x+1)/(x+1))=(x+1)/(x(x+1))=(x+1)/(x^2+x) Multiply the second rational expression by the factors identified in step 6.
7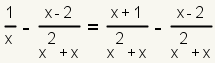1/x - (x-2)/(x^2+x) = (x+1)/(x^2+x) + (x-2)/(x^2+x) Transform the expressions using the multiplied rational expressions.
8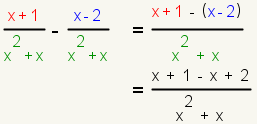((x+2)/(x^2+x)) + ((x-2)/(x^2+x)) = (x + 2 - (x - 2)/(x^2 + x) = (x + 2 - x + 2)/(x^2 + x) Add the numerators, and copy the denominator.
9(x + 2 - x + 2))/(x^2 + x) = 4/(x^2 + x) Simplify the numerator by combining like terms.
10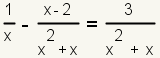1/x + (x-2)/(x^2+x) = 4/(x^2+x) This is the answer.
Example 4 - Subtraction of rational expressions

Multiplication and Division of Rational Expressions

Two or more rational expressions can multiplied. Multiplication and division of rational expressions is similar to multiplication and division of fractions: The steps are:

  • Factor the rational expressions.
  • Cancel common factors.
  • Multiply the numerators.
  • Multiply the denominators.

Example 5

StepEquationDescription
1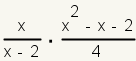(x/(x-2))*((x^2-x-2)/4)This is the expression to multiply
2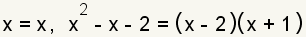x=x, x^2-x-2=(x-2)(x+1)Factor the numerators. This will allow you to more easily cancel common factors.
3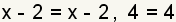x-2=x-2, 4=4Factor the denominators. This will allow you to more easily cancel common factors.
4(x/(x-2))*(((x-2)(x+1))/4) = (x/1)*((x+1)/4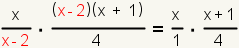)Cancel common factors.
5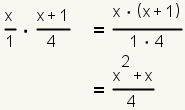(x/1)*((x+1)/4) = (x*(x+1))/(1*4) = (x^2+x)/4Multiply the numerators and multiply the denominators.
6x/(x-2))*((x^2-x-2)/4) = (x^2+x)/4This is the answer.
Example 5 - Multiplication of rational expressions

To divide rational expressions, multiply the divided by the reciprocal of the divisor.

Example 6

StepEquationDescription
1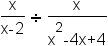(x/(x-2))/(x/(x^2-4x+4))This is the expression to divide.
2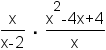(x/(x-2))*((x^2-4x+4)/x)Multiply by the reciprocal of the divisor.
3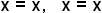x=xFactor the numerators. This will allow you to more easily cancel common factors.
4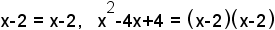x-2=x-2, x^2-4x+4=(x-2)(x-2)Factor the denominators. This will allow you to more easily cancel common factors.
5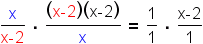(x/(x-2))*((x-2)(x-2))/x) = (1/1)*((x-2)/1)Cancel common factors.
6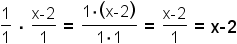(1/1)*((x-2)/1) = (1*1)/((x-2)*1) = 1/(x-2)Multiply the numerators and multiply the denominators.
7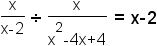(x/(x-2))/(x/(x^2-4x+4)) = 1/(x-2)This is the answer.
Example 6 - Division of rational expressions

References

  1. McAdams, David E.. All Math Words Dictionary, rational expression. 2nd Classroom edition 20150108-4799968. pg 150. Life is a Story Problem LLC. January 8, 2015. Buy the book

Cite this article as:

 McAdams, David E. Rational Expression. 4/30/2019. All Math Words Encyclopedia. Life is a Story Problem LLC. http://www.allmathwords.org/en/r/rationalexpression.html.

Image Credits

Revision History

4/30/2019: Changed equations and expressions to new format. (McAdams, David E.)
12/21/2018: Reviewed and corrected IPA pronunication. (McAdams, David E.)
12/4/2018: Removed broken links, updated license, implemented new markup. (McAdams, David E.)
8/7/2018: Changed vocabulary links to WORDLINK format. (McAdams, David E.)
11/10/2009: Added addition, subtraction, multiplication and division of rational expressions. (McAdams, David E.)
1/20/2009: Initial version. (McAdams, David E.)

All Math Words Encyclopedia is a service of Life is a Story Problem LLC.
Copyright © 2018 Life is a Story Problem LLC. All rights reserved.
This work is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License